The Needle Pulse

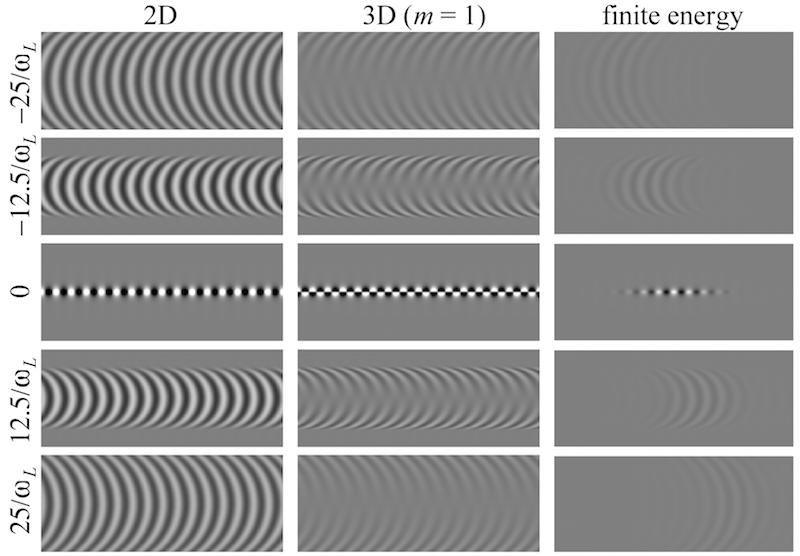
We derived the properties of pulsed solutions to the scalar and vector wave equations composed of plane waves with equal longitudinal spatial frequency. This condition guarantees that, at all times, the field profile is invariant in the longitudinal direction. Particular emphasis is placed on solutions with rotational symmetry. For these solutions, the wave concentrates strongly near the axis at a given time. We provide closed-form expressions for some of these fields, and show that their wavefronts are approximately spherical, and converge to a long and highly concentrated beam along the central axis, hence the name "needle pulse." Solutions carrying orbital and spin angular momenta are also considered for optical analogues, and ultrasound implementations are considered. Read more about Professors Kevin Parker and Miguel Alonso's exciting research here and in the journal articles following.

Journal Articles

  1. The spherical harmonic family of beampatterns
    K. J. Parker and M. A. Alonso
    Acoustics, vol. 4, no. 4 , pp. 958 -966  (2022). View PDF
  2. The nonlinear ultrasound needle pulse
    P. T. Christopher and K. J. Parker
    J Acoust Soc Am, vol. 144, no. 2 , pp. 861 -871  (2018). View PDF
  3. The ultrasound needle pulse
    K. J. Parker, S. Chen, and M. A. Alonso
    IEEE Trans Ultrason Ferroelectr Freq Control, vol. 64, no. 7 , pp. 1045 -1049  (2017). View PDF
  4. Longitudinal iso-phase condition and needle pulses
    K. J. Parker and M. A. Alonso
    Optics Express, vol. 24, no. 55 , pp. 28669 -28677  (2016). View Online